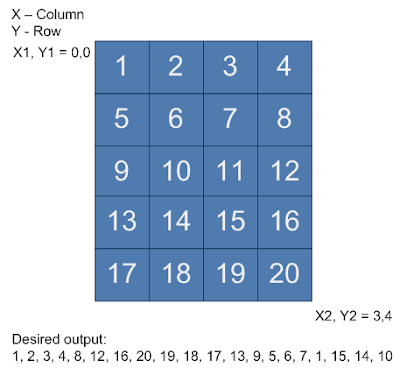
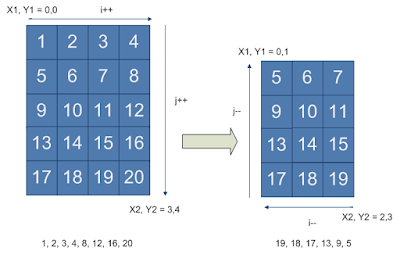
#include
#include
struct node
{
int data;
struct node *next;
};
struct node *head = NULL;
struct node *tail = NULL;
int push(int input)
{
struct node *newnode = (struct node *)malloc(sizeof(struct node));
if(!newnode) return 0;
newnode->data = input;
if(tail) {
tail->next = newnode;
}
newnode->next = NULL;
tail = newnode;
if(!head) head = newnode;
return 1;
}
int display()
{
struct node *current = head;
while(current)
{
printf("%d\n", current->data);
current = current->next;
}
}
int freenodes()
{
struct node *current = head;
if(head) head = head->next;
while(current)
{
free(current);
current = head;
if(head) head = head->next;
}
printf("Linked List Freed\n");
}
int findmaxrepeat()
{
if(!head) return 0;
struct node *current = head->next;
int value = 0;
int count = 0;
int maxvalue = 0;
int maxvaluecnt = 0;
value = head->data; count +=1;
maxvalue = value;
maxvaluecnt = count;
while(current)
{
if(current->data == value) count +=1;
else
{
if(maxvaluecnt < count) { maxvaluecnt = count; maxvalue = value;}
value = current->data; count = 1;
}
current = current->next;
}
return maxvalue;
}
int main()
{
push(1);
push(3);
push(2);
push(3);
push(3);
push(1);
push(1);
push(3);
push(3);
push(1);
push(3);
display();
printf("%d is the maxrepeated value\n", findmaxrepeat());
freenodes();
}
TC O(n)
SC O(1)
Run Here https://ideone.com/qmu7Z